Standard practice for determining the existing load resistance of specific glass units used in buildings, especially in windows, is found in ASTM E1300 [2].
Procedures described in [2] also cover determination of load resistance and maximum lateral deflection for glass types combined in sealed insulating glass units.
Focusing on common practice, the procedures of [2] are applicable for rectangular insulating glass units, simply supported on four sides and exposed to uniform lateral loads of short and long duration.
An approximation of the behavior and probability of fracture of various types of glass combined in sealed IG units as well as a proportional assumption of load sharing between inner and outer lite of double glazed IG units is stated in appendixes X2 and X3 of [2], which is sometimes a conservative approach but not in any case.
Appendix X5 provides an approximate technique to combine various lateral uniform loads of different load duration. However, all assumptions given by [2] are very limited in terms of shape, edge support, load effects and sufficient evaluation of combined types of glass.
For these cases more accurate calculation methods are needed as internal loads have to be taken into account for an IG unit and capable procedures for evaluating separated and combined effects have to be discussed.
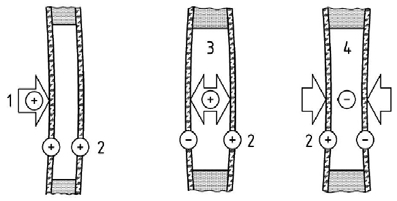
In practice there are additional internal load effects existing if insulating glass units are used. ASTM E1300 is using a load share factor between the lites of an IG unit, but does not address the internal “climatic effect”, deeper described in the next section. Within this section, the rules of load sharing between inner and outer glass shall be presented, first.
Lites of an IG unit are not only linearly bonded around the perimeter. They are also connected by the gas volume enclosed in the hermetically sealed cavity.
This coupling effect causes load sharing between the connected lites.
A simplified approach widely used in engineering practice and mentioned in [2], appendix X3 is determining the correct proportional stiffness of inner and outer glass.
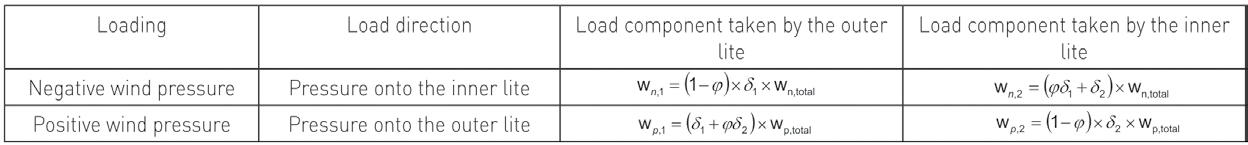
Actually, an accurate approach of load sharing (ref. to Table 2) has to respect the shape and the dimension of the IG unit and the direction of the relevant loading as stated in [3] and [5], too.
Covering all the relevant influences Prof. Feldmeier introduced an insulating glass factor which depends on the length of the shorter glass edge a and the characteristic length a*. a* is representing characteristic properties of the IG unit like aspect ratio Bv (ref. to Table 1), dimension of the hermetically sealed cavity tcavity and stiffness of the two lites (outer lite t1, inner lite t2). The equations to determine these factors are shown in (1).
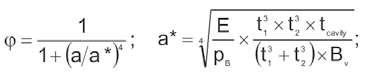
 (1)
(1)
E – Young’s modulus glass – 71 700 MPa
pB – barometric pressure – 100 kPa
Climatic Effects in hermetically sealed units
Insulating glass units are hermetically sealed systems. This means that gas (air or inert gases like argon and krypton) is enclosed in the space between at least two or more lites. It is an isochoric condition. This means that the volume is remaining constant, as shown in (2).
 (2)
(2)
Climatic effects imply internal load effects caused by the expansion of the gas volume. The expansion of the enclosed volume is confined by the inner and the outer lite.
Due to the fact that the lites are not absolutely rigid, the actual state is balanced between an isochoric and an isobaric limit state. The individual loading depends on the external conditions and the geometrical properties.
Geometrical properties are the dimension of the cavity, the dimension of the IG unit and the thickness of the inner and the outer lite. External conditions include
- Variation of atmospheric pressure Δpatm,
- Variation of temperature of the enclosed gas ΔT and
- Variation of elevation ΔH, which can result anincreasing pressure in the cavity.
Variations acting on the glass and the edge seal are also related to changed conditions between production of the IG unit just as the edge seal and conditions after installation or during service life. The relevant isochoric limit state p0 can be determined according to (3).
The geometrical properties are embedded into the insulating glass factor determined in (1). The product of isochoric pressure and insulating glass factor in (4) results in the internal load Pclimatic acting as the maximum climatic load effect on the inner and the outer lite as well as on the edge of the IG unit.
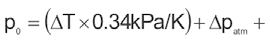
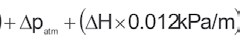 (3)
(3)
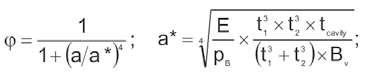 (4)
(4)
While larger IG units are mainly affected by wind loads or other externally imposed loads the impact of climatic effects becomes more and more decisive for smaller and narrow units. In some cases, climatic load effects for the smaller IG units are much higher than wind load impacts relevant for the larger units.
That makes it important to not just consider the largest IG units for a proper glass and secondary seal design. Taking into account climatic load effects and considering smaller IG units is significantly important for the entire glass design, a durable edge sealing system and a final IG configuration which meets all demands on safety and life expectancy.
Allowable Surface stress in accordance with ASTM E1300
The glass failture prediction model (FPM) that serves as a basis for the non-factored load (NFL) charts of ASTM E1300 [2] assumes that the probability of glass breakage is a function of the distribution and severity of stress-raising surface discontinuities and the distribution of surface tensile stresses over the glass area.
If the maximum stress levels on two lites with different dimensions and thicknesses are the same, the lite with the maximum stress distributed over the bigger area is more likely to fail. It is not appropriate to base the structural adequacy of glass used in buildings solely on its modulus of rupture as determined through the testing of small-scale laboratory specimens.
The verification approach of [2] is based on the load resistance (LR) determined as a uniform lateral load. LR is composed of a non-factored load (NFL) representing a maximum applicable load (3-seconds duration, probability of breakage ≤ 0.008, monolithic annealed glass) taking into account surface conditions, glass thickness as well as glass dimensions and of the glass type factor (GTF) representing the appropriate glass type and the relevant load duration.
This procedure becomes more complex if laminated glass (LG), insulating glass (IG) or even the combination of more than one load acting concurrently with different load durations, has to be considered. Depending on the specific case, additional load sharing factors (LS), lite probability factors (p) and combination of load effects and load duration according to [2], X5.1 have to be respected. LR is going to be determined for each lite, while the lower of all load resistance values is decisive. In case of combined loads q and LR are determined for an equivalent 3-seconds duration, shown in (5).
 (5)
(5)
More and more there is demand for designing and verifying glass units of irregular shape or composition, not 4-sided simply supported or even exposed to non-uniform load impacts and load combinations. In these cases, more flexible and accurate calculation techniques such as finite element, finite difference or standard engineering mechanics formulas have to be used to determine maximum surface stress and deflection taking into account specific boundary conditions.
Different from FPM used in [2] most common engineering practices and tools are based on allowable stress design (ASD). Covering design of special glass shapes and loads on an adequate level of safety and confidence ASTM E1300 gives some indications for the approximate maximum surface stress to be used with an independent stress analysis in appendix X6.
Mostly conservative allowable surface stress values for a 3-seconds duration load and a probability of breakage ≤ 0.008 are mentioned in [2], X6.2 with 23.3 MPa (3 380 psi) for annealed float glass, 46.6 MPa (6 750 psi) for heat-strengthened glass and 93.1 MPa (13 500 psi) for fully-tempered glass. X6.3 requires calculating the maximum surface stress in a glass lite using rigorous engineering analysis, which takes into account large defections, like non-linear finite element analysis.
In general, non-linear effects get more significant for the verification of continuously supported glass units the bigger the glass deflection in relation to the glass thickness and the closeness of the aspect ratio to quadratic shape. The calculated surface stress has to be less than the maximum allowable stress.
The maximum allowable surface stress is a function of area (A), load duration in seconds (d), surface flaw parameter (k), probability of breakage (Pb) and an exponent that characterizes the weakening effect due to sub-critical crack growth (n). All of these parameters can be considered as properly represented by the allowable surface stress according to [2], X6.2.
The equation mentioned in X6.2 must be verified in each individual case or within a proper structural design considering different loads and their combination factors. Parameters, which still could be adapted to the specific cases and glass types are the load duration (d) and the exponent n differentiated in [8], Note 2 for different glass types, using n = 16 for annealed float glass, n = 32 for heat-strengthened glass and n = 48 for fully-tempered glass.
Another important point is a suitable evaluation of fritted glass. [8], Note 1 gives a general recommendation for disruptive surface treatments stating an allowable stress reduction factor of 0.5 but with a remarks to consult the glass manufacturer. [9] summarizes a useful study. Based on its conclusions an allowable stress reduction factor of 0.6 is sufficient and reliable.
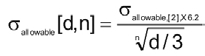 (6)
(6)
Representing the effective conditions, always the minimum glass thickness in accordance with ASTM C1036 is relevant. In case of a European glass manufacturer or float glass supplier the minimum glass thickness according to EN 572 should be taken into account.
For annealed glass, the assumed values for Young´s modulus and Poisson’s ratio are 71.7 GPa (10.4e6 psi) and 0.22, respectively. Based on [2] the PVB interlayer is allowed to be considered with a shear modulus of 0.40 MPa at temperatures up to +50 °C (122 °F) and for 3-seconds load duration.
Combination of relevant loads
ASCE Code 07 [4] is the basic rule for minimum design loads and relevant load combinations in buildings and other structures. The challenge is to define an approach for a proper IG unit design respecting these rules and basic design principles as well as recognizing that both wind loads and climatic effects are leading impacts for capable dimensioning of glass thickness and secondary seal bite.
Actually, climatic conditions, alternating temperatures and different elevations of production site and installation site create expansion or contraction of the enclosed gas space, an effect that is not specifically described in [4].
But assuming that [4] is defining the combination of permanent loads (named as dead load), variable loads (mainly wind load) and self-straining loads (put as systemimplemented load, like climatic effects, only affecting the IG unit itself ), we can split the climatic effect summarized in equation (3) into different components differentiated regarding load duration and combined action.
While effects caused by the difference of elevation ΔH separately create a permanent impact, the combined action of ΔH, temperature difference Δt and difference of atmospheric pressure Δpatm is an effect alternating during the course of a day or the combination of climatic effect and wind is in total a short-term combination.
Furthermore, [4], section 2.4.4 states that it’s unlikely that the maximum effect of selfstraining loads occurs simultaneously with the maximum effect of other variable loads. A combination with 0.75 of the maximum effects is recommended. Table 5 takes into account all summarized aspects for a proper combination of wind load and climatic effects in vertically installed IG units.
Case study: 33 Tehama,
San Francisco, CA, USA
The approach described above has been used for verification of glass design in several projects in the US. The following project helps to compare the normal procedure of ASTM E1300 [2] and an allowable stress design including combined action of wind load and climatic effects.
Relevant for the glass design of the project was not just the estimation of wind loads expected for different zones of the building façade but also a comprehensive thermal analysis including thermal glass stress analysis, calculation of the maximum temperatures of glass components and primary seal as well as the minimum and maximum temperature of the enclosed gas space. These temperatures are basically needed for calculating expected climatic effects.
33 Tehama Tower in San Francisco is a 35-story apartment high-rise building under construction. Its completion is expected in 2017. Due the thermal requirements, the spandrel units were identified as IG units with very high air space temperatures. As mentioned above, small and narrow units can create very high climatic effects. For typical spandrel units one has the accumulation of both extraordinary high temperature and inappropriate small glass dimensions.
The units of GD-6, as a best practice example, were considered in a smaller dimension 100% utilized by climatic effects and in a maximum dimension showing 27% utilization due to the impact of negative wind pressure or even 42% utilization caused by positive wind pressure creating the maximum surface stress on the fitted (disruptively treated) surface of the inner lite. While bigger units are mainly restricted by limitation of deflection, the huge utilization of smaller units caused by climatic effects isn’t recognized by the standard procedure of [2].
Boundary conditions taken into account for glass verification of the spandrel units GD-6:
- Production site (Lauenfoerde, Germany):
97 m [318 ft] a.s.l.
- Installation site (San Francisco, CA, US):
5 m [16 ft] a.s.l.
- Installation height: 123 m [420 ft]
- GD-6: Spandrel unit, insulating glass make-up
--– Outer lite: 6 mm [1/4 in.] Planibel Clearlite ipasol
Neutral 48/27 # 2, fully tempered & heat
soaked
--– Space: 20 mm [3/4 in.] air, aluminum spacer,
black
--– Inner lite: 6 mm [1/4 in.] Planibel Clearlite, fritted,
RAL 7035 # 4, fully tempered & heat soaked
- Dimensions (width x height)
--– Smaller span: 997 mm x 391 mm [39.3 in. x 15.4 in.]
--– Maximum span: 1505 mm x 3452 mm
[59.3 in. x 135.9 in.]
- Wind load (3 sec): -2.155 kPa / +1.915 kPa
[-45 psf / +40 psf ]
- Continuously simply supported
- Internal shadow box make up
--– Internal backup: 63 mm [2 ½ in.] distance, air, not ventilated
--– Insulation: 50 mm [2 in.], R = 1,43 m2K/W
--– Steel back pan: 1 mm [1/32 in.]
--– 200 mm [7 7/8 in.] air, not ventilated,
R = 0.18 m2K/W acc. to ISO 6946
--– 20 mm [3/4 in.] sub ceiling or floor,
R = 0.1 m2K/W
- Isochoric pressure
--– Climatic effects, summer
(ΔTcavity ≤ 80K; Δpatm ≥ -2kPa;
ΔHaltitude ≤ 30m): +29.6 kPa [+618 psf ]
--– Climatic effects, winter
(ΔTcavity ≥ -24K; Δpatm ≤ 4kPa;
ΔHaltitude ≥ 15m): -12.0 kPa [-251 psf ] |
Table 5. Load combinations representative for proper design of vertical insulating glass units kliknij na tabele aby powiekszyć



Fig. 2. Project renderings (© Hines Constructions & Invesco)
Table 6. Maximum surface stress and deflection for load cases of Table 5. The first table shows the results for a linear calculation and the second one for a non-linear calculation based on a Finite Element Analysis. Grey mark indicates the decisive load case kliknij na tabele aby powiekszyć

ASTM E1300 [2] is a simplified concept. Direct comparison of values determined according to [2] with values from a non-linear finite elements analysis is not reliable. Producing a direct comparison between failure prediction model FPM and allowable stress design ASD the type factors for insulating glass stated in [2], X2.2 were neglected. Furthermore, any disruptive effect of the ceramic frit was not taken into account, as Table 7 is a comparison of concepts but does not represent any verification. Wind pressure and climatic effects were evaluated separately.
In step 1 maximum surface stress of the glass units was calculated on a single glass unit respecting the load sharing factors of [2]. In step 2 the more accurate load sharing concept of Table 2 was applied to the determination of load resistance LR according to [2].
Based on this modification a comparison with the simulation of a full IG model was more feasible. Additionally, only minimum glass thickness according to ASTM C1036 was used for comparing the results at similar conditions.
Table 7 shows a good approximation of utilization determined according to FPM and ASD. In general, a more accurate concept of load sharing factors, as given in Table 2 and shown in step 2, is advisable for smaller units.
For bigger units a significant influence of surface area on the load resistance is considered in the FPM of [2] but neglected for the allowable surface stress values according to [2], X6.2. This could have an impact on design and resistance of larger annealed glass lites.
For commercial façade units and SSG application it is common to use tempered or heat-strengthened glass so glass dimensions or accepted loads are mainly limited by allowable deflection and the utilization of allowable surface stress and usually far away from 100 %.
In conclusion, allowable surface stress values defined in [8], X6.2 are conservative for smaller IG units and sufficient for larger ones. But a suitable are appropriate limitation of glass deflection is an important design condition, too.
Table 7. Comparison of FPM concept of ASTM E1300 [2] and ASD concept based on a non-linear finite elements analysis for wind load kliknij na tabele aby powiekszyć
Conclusion
The above-discussed concept and the presented project example show that “Allowable Stress Design” is a useful and suitable practice for finding and verifying a capable glass design as well as including much more significant boundary conditions than provided by the standard procedure used in ASTM E1300 [2].
Important considerations for proper design are not just a suitable link between FPM and ASD, but also a reliable concept of combining different loads with different load durations.
The draft given in Table 5 is specifically considering load effects on vertical IG units. Essential for proper and sustainable design of IG units is an appropriate concept for determining climatic effects caused by the interaction of the enclosed gas space and external environment as well as respecting effective load sharing between inner and outer lites of a double glazed unit.
Here, ASTM E1300 [2] shows some inadequacy, especially regarding smaller and midsize glass dimensions, which can be heavily affected by clima-tic effects and which clearly show a load sharing behavior different from the simplified approach of [2]. For larger glass units, especially those composed of tempered and heat-strengthened glass, the verification based on ASD should be completed by evaluation and limitation of maximum glass deflection.
It should be mentioned that in the European design codes are based on the principle of partial safety specific combination factors. The limit state design approach requires taking into account safety and combination factors for the values of applied stress as well as for the resistance value of the glass product. Both safety factors for the stress and resistance value are separated and represent the specific statistical deviation for loading and material.
Regardless from other international glass codes, this paper describes an approach keeping the systematic of the existing North American standards ASTM E1300 and ASCE 07.
Thanks also to Permasteelisa North America regarding their expert support and their support regarding the case study, as they have done the curtain wall cladding.
The article is based on the lecture presented at the GLASS PERFORMANCE DAYS 2017 Conference, which took place on June 28-30, 2017 in Tampere, Finland
(...)











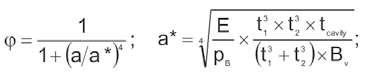
 (1)
(1)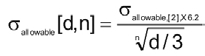 (6)
(6)


























